From Wikipedia, the free encyclopedia
«i (number)» redirects here. For internet numbers, see i-number.
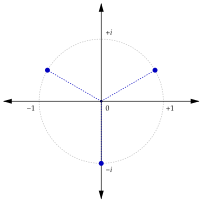
i in the complex or Cartesian plane. Real numbers lie on the horizontal axis, and imaginary numbers lie on the vertical axis.
The imaginary unit or unit imaginary number (i) is a solution to the quadratic equation 

Imaginary numbers are an important mathematical concept; they extend the real number system 

There are two complex square roots of −1: i and 
In contexts in which use of the letter i is ambiguous or problematic, the letter j is sometimes used instead. For example, in electrical engineering and control systems engineering, the imaginary unit is normally denoted by j instead of i, because i is commonly used to denote electric current.[1]
Definition[edit]
| The powers of i return cyclic values: |
|---|
 (repeats the pattern (repeats the patternfrom bold blue area) |

|

|

|

|

|

|

|

|

|

|
 (repeats the pattern (repeats the patternfrom bold blue area) |
The imaginary number i is defined solely by the property that its square is −1:
With i defined this way, it follows directly from algebra that i and 
Although the construction is called «imaginary», and although the concept of an imaginary number may be intuitively more difficult to grasp than that of a real number, the construction is valid from a mathematical standpoint. Real number operations can be extended to imaginary and complex numbers, by treating i as an unknown quantity while manipulating an expression (and using the definition to replace any occurrence of 

or, equivalently,
Similarly, as with any non-zero real number:
As a complex number, i can be represented in rectangular form as 



i vs. −i[edit]
Being a quadratic polynomial with no multiple root, the defining equation 






In fact, if all mathematical textbooks and published literature referring to imaginary or complex numbers were to be rewritten with 




The issue can be a subtle one. One way of articulating the situation is that although the complex field is unique (as an extension of the real numbers) up to isomorphism, it is not unique up to a unique isomorphism. Indeed, there are two field automorphisms of C that keep each real number fixed, namely the identity and complex conjugation. For more on this general phenomenon, see Galois group.
Matrices[edit]
Some imaginary units correspond to points ( x, y ) on the hyperbola xy = −1.
Using the concepts of matrices and matrix multiplication, imaginary units can be represented in linear algebra. The value of 1 is represented by an identity matrix I and the value of i is represented by any matrix J satisfying J2 = −I. A typical choice is
More generally, a real-valued 2 × 2 matrix J satisfies J2 = −I if and only if J has a matrix trace of zero and a matrix determinant of one, so J can be chosen to be
whenever −z2 − xy = 1. The product xy is negative because xy = −(1 + z2); thus, the points (x, y) lie on hyperbolas determined by z in quadrant II or IV.
The usual rules of complex number mathematics work with these matrices because I × I = I, I × J = J, J × I = J, and J × J = −I. For example,
Proper use[edit]
The imaginary unit is sometimes written 

Similarly:
Generally, the calculation rules
and
are guaranteed to be valid for real, positive values of a and b only.[5][6][7]
When a or b is real but negative, these problems can be avoided by writing and manipulating expressions like 

Properties[edit]
Square roots[edit]
The two square roots of i in the complex plane
The three cube roots of i in the complex plane
Just like all nonzero complex numbers, i has two square roots: they are[a]
Indeed, squaring both expressions yields:
Using the radical sign for the principal square root, we get:
Cube roots[edit]
The three cube roots of i are:[9]
and
Similar to all the roots of 1, all the roots of i are the vertices of regular polygons, which are inscribed within the unit circle in the complex plane.
Multiplication and division[edit]
Multiplying a complex number by i gives:
(This is equivalent to a 90° counter-clockwise rotation of a vector about the origin in the complex plane.)
Dividing by i is equivalent to multiplying by the reciprocal of i:
Using this identity to generalize division by i to all complex numbers gives:
(This is equivalent to a 90° clockwise rotation of a vector about the origin in the complex plane.)
Powers[edit]
The powers of i repeat in a cycle expressible with the following pattern, where n is any integer:
This leads to the conclusion that
where mod represents the modulo operation. Equivalently:
Although we do not give the details here, if one chooses branch cuts and principal values to support it then this last equation would apply to all complex values of n.[citation needed]
i raised to the power of i[edit]
Making use of Euler’s formula, 
for any integer k. A common principal value corresponds to 

Factorial[edit]
The factorial of the imaginary unit i is most often given in terms of the gamma function evaluated at 
[15]
The magnitude of this number is
[16]
while its argument is
[17]
Other operations[edit]
Many mathematical operations that can be carried out with real numbers can also be carried out with i, such as exponentiation, roots, logarithms, and trigonometric functions. The following functions are well-defined, single-valued functions when x is a positive real number.
A number raised to the ni power is:
The nith root of a number is:
The cosine of ni is:
which is a real number when n is a real number.
The sine of ni is:
which is a purely imaginary number when n is a real number.
In contrast, many functions involving i, including those that depend upon log i or the logarithm of another complex number, are complex multi-valued functions, with different values on different branches of the Riemann surface the function is defined on.[18] For example, if one chooses any branch where log i = πi/2 then one can write
when x is a positive real number. When x is not a positive real number in the above formulas then one must precisely specify the branch to get a single-valued function; see complex logarithm.
History[edit]
Designating square roots of negative numbers as «imaginary» is generally credited to René Descartes, and Isaac Newton used the term as early as 1670.[19][20] The i notation was introduced by Leonhard Euler.[21]
See also[edit]
- Euler’s identity
- Mathematical constant
- Multiplicity (mathematics)
- Root of unity
- Unit complex number
Notes[edit]
- ^ To find such a number, one can solve the equation
where x and y are real parameters to be determined, or equivalently
Because the real and imaginary parts are always separate, we regroup the terms,
By equating coefficients, separating the real part and imaginary part, we get a system of two equations:
Substituting
into the first equation, we get
Because x is a real number, this equation has two real solutions for x:
and
. Substituting either of these results into the equation
in turn, we will get the corresponding result for y. Thus, the square roots of i are the numbers
and
.[8]
References[edit]
- ^ Boas, Mary L. (2006). Mathematical Methods in the Physical Sciences (3rd ed.). New York [u.a.]: Wiley. p. 49. ISBN 0-471-19826-9.
- ^ a b Weisstein, Eric W. «Imaginary Unit». mathworld.wolfram.com. Retrieved 10 August 2020.
- ^ Doxiadēs, Apostolos K.; Mazur, Barry (2012). Circles Disturbed: The interplay of mathematics and narrative (illustrated ed.). Princeton University Press. p. 225. ISBN 978-0-691-14904-2 – via Google Books.
- ^ Bunch, Bryan (2012). Mathematical Fallacies and Paradoxes (illustrated ed.). Courier Corporation. p. 31-34. ISBN 978-0-486-13793-3 – via Google Books.
- ^ Kramer, Arthur (2012). Math for Electricity & Electronics (4th ed.). Cengage Learning. p. 81. ISBN 978-1-133-70753-0 – via Google Books.
- ^ Picciotto, Henri; Wah, Anita (1994). Algebra: Themes, tools, concepts (Teachers’ ed.). Henri Picciotto. p. 424. ISBN 978-1-56107-252-1 – via Google Books.
- ^ Nahin, Paul J. (2010). An Imaginary Tale: The story of «i» [the square root of minus one]. Princeton University Press. p. 12. ISBN 978-1-4008-3029-9 – via Google Books.
- ^ «What is the square root of i ?». University of Toronto Mathematics Network. Retrieved 26 March 2007.
- ^ Zill, Dennis G.; Shanahan, Patrick D. (2003). A first course in complex analysis with applications. Boston: Jones and Bartlett. pp. 24–25. ISBN 0-7637-1437-2. OCLC 50495529.
- ^ Wells, David (1997) [1986]. The Penguin Dictionary of Curious and Interesting Numbers (revised ed.). UK: Penguin Books. p. 26. ISBN 0-14-026149-4.
- ^ Sloane, N. J. A. (ed.). «Sequence A049006 (Decimal expansion of i^i = exp(-Pi/2))». The On-Line Encyclopedia of Integer Sequences. OEIS Foundation.
- ^ Sloane, N. J. A. (ed.). «Sequence A212879 (Decimal expansion of the absolute value of i!)». The On-Line Encyclopedia of Integer Sequences. OEIS Foundation.
- ^ Ivan, M.; Thornber, N.; Kouba, O.; Constales, D. (2013). «Arggh! Eye factorial . . . Arg(i!)». American Mathematical Monthly. 120: 662–665. doi:10.4169/amer.math.monthly.120.07.660. S2CID 24405635.
- ^ Finch, S. (3 November 2022). «Errata and Addenda to Mathematical Constants«. arXiv:2001.00578 [math.HO].
- ^ Sloane, N. J. A. (ed.). «Sequence A212877 (Decimal expansion of the real part of i!)». The On-Line Encyclopedia of Integer Sequences. OEIS Foundation.Sloane, N. J. A. (ed.). «Sequence A212878 (Decimal expansion of the negated imaginary part of i!)». The On-Line Encyclopedia of Integer Sequences. OEIS Foundation.
- ^ Sloane, N. J. A. (ed.). «Sequence A212879 (Decimal expansion of the absolute value of i!)». The On-Line Encyclopedia of Integer Sequences. OEIS Foundation.
- ^ Sloane, N. J. A. (ed.). «Sequence A212880 (Decimal expansion of the negated argument of i!)». The On-Line Encyclopedia of Integer Sequences. OEIS Foundation.
- ^ Gbur, Greg (2011). Mathematical Methods for Optical Physics and Engineering. Cambridge, U.K.: Cambridge University Press. pp. 278–284. ISBN 978-0-511-91510-9. OCLC 704518582.
- ^ Silver, Daniel S. (November–December 2017). «The New Language of Mathematics». American Scientist. 105 (6): 364–371. doi:10.1511/2017.105.6.364.
- ^ «imaginary number». Oxford English Dictionary (Online ed.). Oxford University Press. (Subscription or participating institution membership required.)
- ^ Boyer, Carl B.; Merzbach, Uta C. (1991). A History of Mathematics. John Wiley & Sons. pp. 439–445. ISBN 978-0-471-54397-8.
Further reading[edit]
- Nahin, Paul J. (1998). An Imaginary Tale: The story of i [the square root of minus one]. Chichester: Princeton University Press. ISBN 0-691-02795-1 – via Archive.org.
External links[edit]
- Euler, Leonhard. «Imaginary Roots of Polynomials». at «Convergence». mathdl.maa.org. Mathematical Association of America. Archived from the original on 13 July 2007.
From Wikipedia, the free encyclopedia
«i (number)» redirects here. For internet numbers, see i-number.
i in the complex or Cartesian plane. Real numbers lie on the horizontal axis, and imaginary numbers lie on the vertical axis.
The imaginary unit or unit imaginary number (i) is a solution to the quadratic equation 

Imaginary numbers are an important mathematical concept; they extend the real number system 

There are two complex square roots of −1: i and 
In contexts in which use of the letter i is ambiguous or problematic, the letter j is sometimes used instead. For example, in electrical engineering and control systems engineering, the imaginary unit is normally denoted by j instead of i, because i is commonly used to denote electric current.[1]
Definition[edit]
| The powers of i return cyclic values: |
|---|
 (repeats the pattern (repeats the patternfrom bold blue area) |

|

|

|

|

|

|

|

|

|

|
 (repeats the pattern (repeats the patternfrom bold blue area) |
The imaginary number i is defined solely by the property that its square is −1:
With i defined this way, it follows directly from algebra that i and 
Although the construction is called «imaginary», and although the concept of an imaginary number may be intuitively more difficult to grasp than that of a real number, the construction is valid from a mathematical standpoint. Real number operations can be extended to imaginary and complex numbers, by treating i as an unknown quantity while manipulating an expression (and using the definition to replace any occurrence of 

or, equivalently,
Similarly, as with any non-zero real number:
As a complex number, i can be represented in rectangular form as 



i vs. −i[edit]
Being a quadratic polynomial with no multiple root, the defining equation 






In fact, if all mathematical textbooks and published literature referring to imaginary or complex numbers were to be rewritten with 




The issue can be a subtle one. One way of articulating the situation is that although the complex field is unique (as an extension of the real numbers) up to isomorphism, it is not unique up to a unique isomorphism. Indeed, there are two field automorphisms of C that keep each real number fixed, namely the identity and complex conjugation. For more on this general phenomenon, see Galois group.
Matrices[edit]
Some imaginary units correspond to points ( x, y ) on the hyperbola xy = −1.
Using the concepts of matrices and matrix multiplication, imaginary units can be represented in linear algebra. The value of 1 is represented by an identity matrix I and the value of i is represented by any matrix J satisfying J2 = −I. A typical choice is
More generally, a real-valued 2 × 2 matrix J satisfies J2 = −I if and only if J has a matrix trace of zero and a matrix determinant of one, so J can be chosen to be
whenever −z2 − xy = 1. The product xy is negative because xy = −(1 + z2); thus, the points (x, y) lie on hyperbolas determined by z in quadrant II or IV.
The usual rules of complex number mathematics work with these matrices because I × I = I, I × J = J, J × I = J, and J × J = −I. For example,
Proper use[edit]
The imaginary unit is sometimes written 

Similarly:
Generally, the calculation rules
and
are guaranteed to be valid for real, positive values of a and b only.[5][6][7]
When a or b is real but negative, these problems can be avoided by writing and manipulating expressions like 

Properties[edit]
Square roots[edit]
The two square roots of i in the complex plane
The three cube roots of i in the complex plane
Just like all nonzero complex numbers, i has two square roots: they are[a]
Indeed, squaring both expressions yields:
Using the radical sign for the principal square root, we get:
Cube roots[edit]
The three cube roots of i are:[9]
and
Similar to all the roots of 1, all the roots of i are the vertices of regular polygons, which are inscribed within the unit circle in the complex plane.
Multiplication and division[edit]
Multiplying a complex number by i gives:
(This is equivalent to a 90° counter-clockwise rotation of a vector about the origin in the complex plane.)
Dividing by i is equivalent to multiplying by the reciprocal of i:
Using this identity to generalize division by i to all complex numbers gives:
(This is equivalent to a 90° clockwise rotation of a vector about the origin in the complex plane.)
Powers[edit]
The powers of i repeat in a cycle expressible with the following pattern, where n is any integer:
This leads to the conclusion that
where mod represents the modulo operation. Equivalently:
Although we do not give the details here, if one chooses branch cuts and principal values to support it then this last equation would apply to all complex values of n.[citation needed]
i raised to the power of i[edit]
Making use of Euler’s formula, 
for any integer k. A common principal value corresponds to 

Factorial[edit]
The factorial of the imaginary unit i is most often given in terms of the gamma function evaluated at 
[15]
The magnitude of this number is
[16]
while its argument is
[17]
Other operations[edit]
Many mathematical operations that can be carried out with real numbers can also be carried out with i, such as exponentiation, roots, logarithms, and trigonometric functions. The following functions are well-defined, single-valued functions when x is a positive real number.
A number raised to the ni power is:
The nith root of a number is:
The cosine of ni is:
which is a real number when n is a real number.
The sine of ni is:
which is a purely imaginary number when n is a real number.
In contrast, many functions involving i, including those that depend upon log i or the logarithm of another complex number, are complex multi-valued functions, with different values on different branches of the Riemann surface the function is defined on.[18] For example, if one chooses any branch where log i = πi/2 then one can write
when x is a positive real number. When x is not a positive real number in the above formulas then one must precisely specify the branch to get a single-valued function; see complex logarithm.
History[edit]
Designating square roots of negative numbers as «imaginary» is generally credited to René Descartes, and Isaac Newton used the term as early as 1670.[19][20] The i notation was introduced by Leonhard Euler.[21]
See also[edit]
- Euler’s identity
- Mathematical constant
- Multiplicity (mathematics)
- Root of unity
- Unit complex number
Notes[edit]
- ^ To find such a number, one can solve the equation
where x and y are real parameters to be determined, or equivalently
Because the real and imaginary parts are always separate, we regroup the terms,
By equating coefficients, separating the real part and imaginary part, we get a system of two equations:
Substituting
into the first equation, we get
Because x is a real number, this equation has two real solutions for x:
and
. Substituting either of these results into the equation
in turn, we will get the corresponding result for y. Thus, the square roots of i are the numbers
and
.[8]
References[edit]
- ^ Boas, Mary L. (2006). Mathematical Methods in the Physical Sciences (3rd ed.). New York [u.a.]: Wiley. p. 49. ISBN 0-471-19826-9.
- ^ a b Weisstein, Eric W. «Imaginary Unit». mathworld.wolfram.com. Retrieved 10 August 2020.
- ^ Doxiadēs, Apostolos K.; Mazur, Barry (2012). Circles Disturbed: The interplay of mathematics and narrative (illustrated ed.). Princeton University Press. p. 225. ISBN 978-0-691-14904-2 – via Google Books.
- ^ Bunch, Bryan (2012). Mathematical Fallacies and Paradoxes (illustrated ed.). Courier Corporation. p. 31-34. ISBN 978-0-486-13793-3 – via Google Books.
- ^ Kramer, Arthur (2012). Math for Electricity & Electronics (4th ed.). Cengage Learning. p. 81. ISBN 978-1-133-70753-0 – via Google Books.
- ^ Picciotto, Henri; Wah, Anita (1994). Algebra: Themes, tools, concepts (Teachers’ ed.). Henri Picciotto. p. 424. ISBN 978-1-56107-252-1 – via Google Books.
- ^ Nahin, Paul J. (2010). An Imaginary Tale: The story of «i» [the square root of minus one]. Princeton University Press. p. 12. ISBN 978-1-4008-3029-9 – via Google Books.
- ^ «What is the square root of i ?». University of Toronto Mathematics Network. Retrieved 26 March 2007.
- ^ Zill, Dennis G.; Shanahan, Patrick D. (2003). A first course in complex analysis with applications. Boston: Jones and Bartlett. pp. 24–25. ISBN 0-7637-1437-2. OCLC 50495529.
- ^ Wells, David (1997) [1986]. The Penguin Dictionary of Curious and Interesting Numbers (revised ed.). UK: Penguin Books. p. 26. ISBN 0-14-026149-4.
- ^ Sloane, N. J. A. (ed.). «Sequence A049006 (Decimal expansion of i^i = exp(-Pi/2))». The On-Line Encyclopedia of Integer Sequences. OEIS Foundation.
- ^ Sloane, N. J. A. (ed.). «Sequence A212879 (Decimal expansion of the absolute value of i!)». The On-Line Encyclopedia of Integer Sequences. OEIS Foundation.
- ^ Ivan, M.; Thornber, N.; Kouba, O.; Constales, D. (2013). «Arggh! Eye factorial . . . Arg(i!)». American Mathematical Monthly. 120: 662–665. doi:10.4169/amer.math.monthly.120.07.660. S2CID 24405635.
- ^ Finch, S. (3 November 2022). «Errata and Addenda to Mathematical Constants«. arXiv:2001.00578 [math.HO].
- ^ Sloane, N. J. A. (ed.). «Sequence A212877 (Decimal expansion of the real part of i!)». The On-Line Encyclopedia of Integer Sequences. OEIS Foundation.Sloane, N. J. A. (ed.). «Sequence A212878 (Decimal expansion of the negated imaginary part of i!)». The On-Line Encyclopedia of Integer Sequences. OEIS Foundation.
- ^ Sloane, N. J. A. (ed.). «Sequence A212879 (Decimal expansion of the absolute value of i!)». The On-Line Encyclopedia of Integer Sequences. OEIS Foundation.
- ^ Sloane, N. J. A. (ed.). «Sequence A212880 (Decimal expansion of the negated argument of i!)». The On-Line Encyclopedia of Integer Sequences. OEIS Foundation.
- ^ Gbur, Greg (2011). Mathematical Methods for Optical Physics and Engineering. Cambridge, U.K.: Cambridge University Press. pp. 278–284. ISBN 978-0-511-91510-9. OCLC 704518582.
- ^ Silver, Daniel S. (November–December 2017). «The New Language of Mathematics». American Scientist. 105 (6): 364–371. doi:10.1511/2017.105.6.364.
- ^ «imaginary number». Oxford English Dictionary (Online ed.). Oxford University Press. (Subscription or participating institution membership required.)
- ^ Boyer, Carl B.; Merzbach, Uta C. (1991). A History of Mathematics. John Wiley & Sons. pp. 439–445. ISBN 978-0-471-54397-8.
Further reading[edit]
- Nahin, Paul J. (1998). An Imaginary Tale: The story of i [the square root of minus one]. Chichester: Princeton University Press. ISBN 0-691-02795-1 – via Archive.org.
External links[edit]
- Euler, Leonhard. «Imaginary Roots of Polynomials». at «Convergence». mathdl.maa.org. Mathematical Association of America. Archived from the original on 13 July 2007.
Мни́мая едини́ца — комплексное число, квадрат которого равен [math]displaystyle{ -1 }[/math]. В математике, физике мнимая единица обозначается латинской буквой [math]displaystyle{ i }[/math] (в электротехнике: [math]displaystyle{ j }[/math])[1][2].
Введение мнимой единицы позволяет расширить поле вещественных чисел до поля комплексных чисел. Одной из причин введения мнимой единицы является то, что не каждое полиномиальное уравнение [math]displaystyle{ f(x)=0 }[/math] с вещественными коэффициентами имеет решения в поле вещественных чисел. Так, уравнение [math]displaystyle{ x^2 + 1 = 0 }[/math] не имеет вещественных корней. Однако оказывается, что любое полиномиальное уравнение с комплексными коэффициентами имеет комплексное решение — об этом говорит основная теорема алгебры. Существуют и другие области, в которых комплексные числа приносят большую пользу.
Исторически мнимая единица сначала была введена для решения вещественного кубического уравнения: при наличии трёх вещественных корней для получения двух из них формула Кардано требовала извлечения квадратных корней из отрицательных чисел.
Вплоть до конца XIX века наряду с символом [math]displaystyle{ i }[/math] использовалось обозначение [math]displaystyle{ sqrt{-1}, }[/math] однако современные источники предписывают во избежание ошибок под знаком радикала помещать только неотрицательные выражения[3][4]. Более того, помимо мнимой единицы, существует ещё одно комплексное число, квадрат которого равен [math]displaystyle{ -1, }[/math] — число [math]displaystyle{ -i, }[/math] в паре с которым мнимая единица составляет следующие свойства:
- числа i и −i являются одновременно противоположными и обратными: последнее верно потому, что произведение этих чисел равно 1;
- i и −i комплексно сопряжены, так что их сумма (ноль) и произведение (единица) вещественны одновременно (свойства сопряжённых чисел).
Термин «мнимая единица» может употребляться не только для комплексных чисел, но и для их обобщений[⇨].
Степени мнимой единицы
Степени [math]displaystyle{ i }[/math] повторяются в цикле:
- [math]displaystyle{ ldots }[/math]
- [math]displaystyle{ i^{-3} = i }[/math]
- [math]displaystyle{ i^{-2} = -1 }[/math]
- [math]displaystyle{ i^{-1} = -i }[/math]
- [math]displaystyle{ i^0 = 1 }[/math]
- [math]displaystyle{ i^1 = i }[/math]
- [math]displaystyle{ i^2 = -1 }[/math]
- [math]displaystyle{ i^3 = -i }[/math]
- [math]displaystyle{ i^4 = 1 }[/math]
- [math]displaystyle{ ldots }[/math]
что может быть записано для любой степени в виде:
- [math]displaystyle{ i^{4n} = 1 }[/math]
- [math]displaystyle{ i^{4n+1} = i }[/math]
- [math]displaystyle{ i^{4n+2} = -1 }[/math]
- [math]displaystyle{ i^{4n+3} = -i }[/math]
где n — любое целое число.
Отсюда: [math]displaystyle{ i^n = i^{n bmod 4} }[/math], где mod 4 — это остаток от деления на 4.
Возведение в комплексную степень является многозначной функцией. Например, таковой является величина [math]displaystyle{ i^i }[/math], которая представляет бесконечное множество вещественных чисел ([math]displaystyle{ i^i subset R }[/math]):
- [math]displaystyle{ i^i = e^{-left(frac{pi}2 + 2pi kright)}, }[/math] где [math]displaystyle{ kinZ. }[/math]
При [math]displaystyle{ k = 0 }[/math] получаем число [math]displaystyle{ e^{-frac{pi}2} = 0{,}20787957635…, }[/math] соответствующее главному значению аргумента (или главному значению комплексного натурального логарифма) мнимой единицы.
Доказательство
- Представим основание в виде комплексной экспоненты (в этом случае её показателем будет комплексный логарифм):
- [math]displaystyle{ z = i^i = left ( e^{operatorname{Ln} i} right )^i =
left ( e^{ln |i| + i operatorname{Arg} i } right )^i }[/math]
Альтернативным путем является представление основания в показательной форме:
- [math]displaystyle{ z = i^i = left ( |i| e^{i operatorname{Arg} i } right )^i }[/math]
Нетрудно убедиться, что оба полученных выражения тождественно равны.
Найдем модуль и аргумент числа [math]displaystyle{ i }[/math]:
- [math]displaystyle{ i = 0 + 1i = x + yi quad Rightarrow quad x = 0, y = 1 }[/math]
- [math]displaystyle{ |i| = sqrt{x^2 + y^2} = sqrt{0^2 + 1^2} = 1 }[/math]
- [math]displaystyle{ operatorname{Arg} i = varphi + 2 pi k }[/math], где [math]displaystyle{ k in Z }[/math]
- [math]displaystyle{ varphi = lim_{x to +0} left ( operatorname{arctg} frac{y}{x} right ) =
lim_{x to +0} left ( operatorname{arctg} frac{1}{x} right ) =
left [{ color{white} vdots } operatorname{arctg} (+infty) { color{white} vdots } right ] = frac{pi}{2} }[/math] - [math]displaystyle{ operatorname{Arg} i = frac{pi}{2} + 2 pi k }[/math]
Подставим полученные значения для модуля и аргумента в выражение для [math]displaystyle{ z }[/math]:
- [math]displaystyle{ z = left ( e^{ln 1 + i left( frac{pi}{2} + 2 pi k right ) } right )^i =
left ( e^{i left( frac{pi}{2} + 2 pi k right ) } right )^i =
e^{i^2 left( frac{pi}{2} + 2 pi k right ) } =
e^{- left( frac{pi}{2} + 2 pi k right ) } }[/math]
Таким образом, получаем:
- [math]displaystyle{ i^i = e^{- left( frac{pi}{2} + 2 pi k right )} }[/math], где [math]displaystyle{ k in Z }[/math] ∎
И очевидно, что:
- [math]displaystyle{ i^i subset R }[/math]
- Теперь докажем, что число [math]displaystyle{ e^{-frac{pi}{2}} }[/math] является частным значением [math]displaystyle{ i^i }[/math], которое соответствует главному значению аргумента (или главному значению комплексного натурального логарифма) мнимой единицы.
Ранее было найдено главное значение аргумента мнимой единицы (т.е. такое, что попадает в промежуток [math]displaystyle{ (-pi, pi] }[/math]):
- [math]displaystyle{ varphi = frac{pi}{2} in (-pi, pi] }[/math]
Подставляя его вместо [math]displaystyle{ operatorname{Arg} i }[/math] в выражение для [math]displaystyle{ z }[/math], получим искомое частное значение:
- [math]displaystyle{ e^{-varphi} = e^{-frac{pi}{2}} = 0{,}20787957635ldots }[/math] ∎
Также верно, что [math]displaystyle{ (-i)^{(-i)}=i^i }[/math].
Факториал
Факториал мнимой единицы i можно определить как значение гамма-функции от аргумента 1 + i:
- [math]displaystyle{ i! = Gamma(1+i) approx 0.4980 — 0.1549i. }[/math]
Также
- [math]displaystyle{ |i!| = sqrt{pi over sinh(pi)} approx 0.521564…, }[/math][5]
потому что |i!|2 = i! i! = i! (i)! = Γ(1 + i) Γ(1 − i), что по рекуррентному соотношению гамма-функции можно переписать как i Γ(i) Γ(1 − i), а затем по формуле дополнения Эйлера — как iπsin πi = πsinh π.
Корни из мнимой единицы
Корни квадратные из мнимой единицы
Корни кубические из мнимой единицы (вершины треугольника)
В поле комплексных чисел корень n-й степени имеет n значений. На комплексной плоскости корни из мнимой единицы находятся в вершинах правильного n-угольника, вписанного в окружность с единичным радиусом.
- [math]displaystyle{ u_k=cos {frac{{frac{pi}{2}} + 2pi k}{n}} +i sin {frac{{frac{pi}{2}} + 2pi k}{n}}, quad k=0,1,…,n-1 }[/math]
В частности, [math]displaystyle{ {sqrt i} = left{frac{1+i}sqrt2; ~frac{-1-i}sqrt2 right} }[/math] и [math]displaystyle{ {sqrt[3]i} = left{-i;~frac{i+sqrt3}2;~ frac{i-sqrt3}2right}. }[/math]
Также корни из мнимой единицы могут быть представлены в показательном виде:
- [math]displaystyle{ u_k=e^{frac{(frac{pi}{2} + 2pi k) i}{n} }, quad k=0,1,…,n-1. }[/math]
Иные мнимые единицы
В конструкции удвоения по Кэли — Диксону или в рамках алгебры по Клиффорду «мнимых единиц расширения» может быть несколько. Но в этом случае могут возникать делители нуля и иные свойства, отличные от свойств комплексного «i».
Например, в теле кватернионов три антикоммутативных мнимых единицы, а также имеется бесконечно много решений уравнения [math]displaystyle{ x^2 = -1 }[/math].
К вопросу об интерпретации и названии
Гаусс утверждал также, что если бы величины 1, −1 и √−1 назывались соответственно не положительной, отрицательной и мнимой единицей, а прямой, обратной и побочной, то у людей не создавалось бы впечатления, что с этими числами связана какая-то мрачная тайна. По словам Гаусса, геометрическое представление дает истинную метафизику мнимых чисел в новом свете. Именно Гаусс ввел термин «комплексные числа» (в противоположность «мнимым числам» Декарта) и использовал для обозначения √−1 символ i.Морис Клайн, «Математика. Утрата определённости». Глава VII. Нелогичное развитие: серьёзные трудности на пороге XIX в.
Обозначения
Обычное обозначение — [math]displaystyle{ i }[/math], но в электро- и радиотехнике мнимую единицу принято обозначать [math]displaystyle{ j }[/math], чтобы не путать с обозначением мгновенной силы тока: [math]displaystyle{ i = i (t) }[/math].
В языке программирования Python мнимая единица записывается как 1j.
В языке программирования Wolfram Language мнимая единица записывается как 𝕚.
См.также
- Дуальные числа и двойные числа
- Комплексный анализ
- Кватернион
- Гиперкомплексное число
Примечания
- ↑ Комплексное число // Большая российская энциклопедия : [в 35 т.] / гл. ред. Ю. С. Осипов. — М. : Большая российская энциклопедия, 2004—2017.
- ↑ Мнимая единица // Математическая энциклопедия (в 5 томах). — М.: Советская Энциклопедия, 1982. — Т. 3. — С. 708.
- ↑ Зайцев В. В., Рыжков В. В., Сканави М. И. Элементарная математика. Повторительный курс. — Издание третье, стереотипное. — М.: Наука, 1976. — С. 49. — 591 с.
- ↑ Корн Г., Корн Т. Справочник по математике (для научных работников и инженеров). — 2-е изд. — М.: Наука, 1970. — С. 33. — 720 с.
- ↑ «abs(i!) Архивная копия от 6 июля 2015 на Wayback Machine», WolframAlpha.
Ссылки
- Мнимая единица // Большая советская энциклопедия : [в 30 т.] / гл. ред. А. М. Прохоров. — 3-е изд. — М. : Советская энциклопедия, 1969—1978.
Мнимая единица — обычно комплексное число, квадрат которого равен отрицательной единице. Однако возможны и иные варианты: в конструкции удвоения по Кэли—Диксона или в рамках алгебр по Клиффорду.
Содержание
- 1 Для комплексных чисел
- 1.1 Определение
- 1.2 Степени мнимой единицы
- 1.3 Факториал
- 1.4 Корни из мнимой единицы
- 2 Иные мнимые единицы
- 3 См.также
- 4 Ссылки
Для комплексных чисел
В математике, физике мнимая единица обозначается как латинская 

Причиной введения мнимой единицы является то, что не каждое полиномиальное уравнение 

Исторически мнимая единица сначала была введена для решения вещественного кубического уравнения: нередко, при наличии трёх вещественных корней, для получения двух из них формула Кардано требовала брать кубический корень в комплексных числах.
Утверждение, что мнимая единица — это «квадратный корень из −1», не точно: ведь «−1» имеет два квадратных корня, один из которых можно обозначить как «i», а другой как «-i». Какой именно корень принять за мнимую единицу — неважно: все равенства сохранят силу при одновременной замене всех «i» на «-i» и «-i» и на «i». Однако из-за этой двусмысленности, чтобы избежать ошибочных выкладок, не следует применять обозначение для 

Определение
Мнимая единица — это число, квадрат которого равен −1. Т.е. 
или
И тогда его вторым решением уравнения будет 
Степени мнимой единицы
Степени i повторяются в цикле:
Что может быть записано для любой степени в виде:
где n — любое целое число.
Отсюда: 
Число 
[1]
Факториал
Факториал мнимой единицы i можно определить как значение гамма-функции от аргумента 1 + i:
Также,
[2]
Корни из мнимой единицы
В поле комплексных чисел корень n-ой степени имеет n решений. На комплексной плоскости эти корни находятся в вершинах правильного n-угольника, вписанного в окружность с единичным радиусом.
Это следует из формулы Муавра и того, что мнимая единица может быть представлена в тригонометрическом виде:
В частности, 
Также корни мнимой единицы могут быть представлены в показательном виде:
Иные мнимые единицы
В конструкции Кэли — Диксона (или в алгебрах Клиффорда) «мнимых единиц расширения» может быть несколько, и/или их квадрат может быть =«+1» или даже =«0». Но в этом случае могут возникать делители нуля, имеются и иные свойства, отличные от свойств комплексного «i». Например, в теле кватернионов три антикоммутативных мнимых единицы, а также имеется бесконечно много решений уравнения «
См.также
- Дуальные числа и Двойные числа
- Комплексный анализ
- Кватернион
- Гиперкомплексные числа
Ссылки
- ↑ Показательная форма комплексного числа
- ↑ «abs(i!)», WolframAlpha.
- http://slovari.yandex.ru/dict/bse/article/00048/74100.htm
Комплексные числа
1. Понятие мнимой единицы
Допустим,
что существует такое число, квадрат
которого равен – 1. Обозначим это число
буквой i;
тогда можно записать: i2
= – 1.
Число
i
будем называть мнимой
единицей (i
– начальная буква французского слова
imaginaire – «мнимый»), а предыдущее равенство
будем считать определением мнимой
единицы.
Из
этого равенства находим
Введение
мнимой единицы позволяет нам теперь
извлекать корни квадратные из отрицательных
чисел.
Например,

2. Степени мнимой единицы
Рассмотрим
степени мнимой единицы:
i;
i2
= – 1;
i3
= i2*i
= (– 1)i
= – i;
i4
= i3*i
= – i*i
= – i2
= – (– 1) = 1;
i5
= i4*i
= 1*i
= i;
i6
= i5*i
= i*i
= i2
= – 1;
i7
= i6*i
= (– 1)*i
= – i;
i8
= i7*i
= – i*i
= 1;
. . . . . . . . . . . . . . . . . . . . . . . . . .
Если
выписать все значения степеней числа
i,
то мы получим такую последовательность:
i,
– 1, – i,
1, i,
– 1, – i,
1
и т. д. Легко видеть, что значения степеней
числа i
повторяются с периодом, равным 4.
Так,
i
= i,
i2
= – 1, i3
= – i,
i4
= 1, i5
= i,
i6
= – 1, i7
= – i,
i8
= 1, i9
= i,
i10
= – 1, i11
= – i,
i12
= 1.
Таким
образом, если показатель степени числа
i
делится на 4, то значение степени равно
1; если при делении показателя степени
на 4 в остатке получается 1, то значение
степени равно i;
если при делении показателя степени на
4 получается остаток 2, то значение
степени равно – 1; наконец, если при
делении на 4 остаток равен 3, то значение
степени равно – i.
Пользуясь этим, можно вычислять любую
степень числа i.
Пример
1.
Найти: i28;
i33;
i135.
Решение.
Имеем 28
= 4Ч7
(нет остатка); 33
= 4Ч8 + 1; 135 = 4Ч33 + 3.
Соответственно
получим i28
= 1; i33
= i;
i135
= – i.
1–7.
Вычислите:
1.
i66;
i143;
i216;
i137.
2.
i43
+ i48
+ i44
+ i45.
3.
(i36
+ i17)i23.
4.
(i133
+ i115
+ i200
+ i142)(i17
+ i36).
5.
i145
+ i147
+ i264
+ i345
+ i117.
6.
(i13
+ i14
+ i15)i32.
7.
(i64
+ i17
+ i13
+ i82)(i72
– i34).
3. Определение комплексного числа
Мы
знакомы с действительными числами и с
мнимыми единицами. Рассмотрим теперь
числа нового вида.
Определение
1.
Числа вида a
+ bi,
где a
и b
– действительные числа, i – мнимая
единица, будем называть комплексными.
Число
a
будем назвать действительной
частью
комплексного числа, bi
– мнимой
частью
комплексного числа, b
– коэффициентом
при мнимой части.
Возможны случаи, когда действительные
числа a
и b
могут быть равными нулю. Если a
= 0, то комплексное число bi
называется чисто
мнимым.
Если b
= 0, то комплексное число a
+ bi
равно a
и называется действительным.
Если a
= 0 и b
= 0 одновременно, то комплексное число
0 + 0i
равно нулю. Итак, мы получили, что
действительные числа и чисто мнимые
числа представляют собой частные случаи
комплексного числа.
Запись
комплексного числа в виде a
+ bi
называется алгебраической
формой
комплексного числа.
Два
комплексных числа a
+ bi
и c
+ di условились
считать равными
тогда и только тогда, когда в отдельности
равны их действительные части и
коэффициенты при мнимой единице, т. е.
a
+ bi
= c
+ di,
если a
= c
и b
= d.
Пример
2.
Найти x
и y
из равенства:
а)
3y
+ 5xi
= 15 – 7i;
б)
(2x
+ 3y)
+ (x
– y)i
= 7 + 6i.
Решение.
а) Согласно условию равенства комплексных
чисел имеем 3y
= 15, 5x
= – 7. Отсюда
б)
Из условия равенства комплексных чисел
следует
Умножив
второе уравнение на 3 и сложив результат
с первым уравнением, имеем 5x
= 25, т. е. x
= 5. Подставим это значение во второе
уравнение: 5 – y
= 6, откуда y
= – 1. Итак, получаем ответ: x
= 5, y
= – 1.
8–13.
Найдите значения x
и y
из равенств:
8.
7x
+ 5i
= 1 – 10iy.
9.
(2x
+ y)
– i
= 5 + (y
– x)i.
10.
x
+ (3x
– y)i
= 2 – i.
11.
(1
+ 2i)x
+ (3 – 5i)y
= 1 – 3i.
12.
(2 – i)x
+ (1 + i)y
= 5 – i.
13.
(3i
– 1)x
+ (2 – 3i)y
= 2 – 3i.
Соседние файлы в предмете [НЕСОРТИРОВАННОЕ]
- #
- #
- #
- #
- #
- #
- #
- #
- #
- #
- #
Мни́мая едини́ца — комплексное число, квадрат которого равен −1 (минус единице). Термин употребляется также в обобщённом смысле в конструкции удвоения по Кэли — Диксону или в рамках алгебры по Клиффорду.
Содержание
- 1 Для комплексных чисел
- 1.1 Определение
- 1.2 Степени мнимой единицы
- 1.3 Факториал
- 1.4 Корни из мнимой единицы
- 2 Иные мнимые единицы
- 3 К вопросу об интерпретации и названии
- 4 Обозначения
- 5 См.также
- 6 Примечания
- 7 Ссылки
Для комплексных чисел
В математике, физике мнимая единица обозначается как латинская 

Причиной введения мнимой единицы является то, что не каждое полиномиальное уравнение 

Исторически мнимая единица сначала была введена для решения вещественного кубического уравнения: нередко, при наличии трёх вещественных корней, для получения двух из них формула Кардано требовала брать кубический корень в комплексных числах.
Утверждение, что мнимая единица — это «квадратный корень из −1», не совсем точно: ведь «−1» имеет два квадратных корня, один из которых можно обозначить как «i», а другой как «−i». Какой именно корень принять за мнимую единицу — неважно: все равенства сохранят силу при одновременной замене всех «i» на «-i» и «-i» на «i». Однако из-за этой двусмысленности, чтобы избежать ошибочных выкладок, не следует применять обозначение для 

Определение
Мнимая единица — это число, квадрат которого равен −1. Т.е. 
или
И тогда его вторым решением уравнения будет 
Степени мнимой единицы
Степени 
Что может быть записано для любой степени в виде:
где n — любое целое число.
Отсюда:
где mod 4 — это остаток от деления на 4.
Из тождества Эйлера следует, что число 
.
Точнее, в комплексном анализе возведение в степень: 
, где
.
Также верно, что 
Факториал
Факториал мнимой единицы i можно определить как значение гамма-функции от аргумента 1 + i:
Также
[1]
Корни из мнимой единицы
Корни квадратные из мнимой единицы
Корни кубические из мнимой единицы (вершины треугольника)
В поле комплексных чисел корень n-й степени имеет n решений. На комплексной плоскости корни из мнимой единицы находятся в вершинах правильного n-угольника, вписанного в окружность с единичным радиусом.
Это следует из формулы Муавра и того, что мнимая единица может быть представлена в тригонометрическом виде:
В частности, 
![{sqrt[{3}]{i}}=left{-i; {frac {i+{sqrt {3}}}{2}}; {frac {i-{sqrt {3}}}{2}}right}](https://wikimedia.org/api/rest_v1/media/math/render/svg/8928e2fc3cffb989559627bf1d5e185801b76230)
Также корни из мнимой единицы могут быть представлены в показательном виде:
Иные мнимые единицы
В конструкции Кэли — Диксона (или в алгебрах Клиффорда) «мнимых единиц расширения» может быть несколько, и/или их квадрат может быть = «+1» или даже = «0». Но в этом случае могут возникать делители нуля, имеются и иные свойства, отличные от свойств комплексного «i».
Например, в теле кватернионов три антикоммутативных мнимых единицы, а также имеется бесконечно много решений уравнения «
К вопросу об интерпретации и названии
| Гаусс утверждал также, что если бы величины 1, −1 и √−1 назывались соответственно не положительной, отрицательной и мнимой единицей, а прямой, обратной и побочной, то у людей не создавалось бы впечатления, что с этими числами связана какая-то мрачная тайна. По словам Гаусса, геометрическое представление дает истинную метафизику мнимых чисел в новом свете. Именно Гаусс ввел термин «комплексные числа» (в противоположность «мнимым числам» Декарта) и использовал для обозначения √−1 символ i.
Морис Клайн, «Математика. Утрата определённости». Глава VII. Нелогичное развитие: серьёзные трудности на пороге XIX в. |
Обозначения
Обычное обозначение 


В языке программирования Python мнимая единица записывается как 1j.
См.также
- Дуальные числа и Двойные числа
- Комплексный анализ
- Кватернион
- Гиперкомплексное число
Примечания
- ↑ «abs(i!)», WolframAlpha.
Ссылки
- Мнимая единица // Большая советская энциклопедия : [в 30 т.] / гл. ред. А. М. Прохоров. — 3-е изд. — М. : Советская энциклопедия, 1969—1978.
Мни́мая едини́ца — комплексное число, квадрат которого равен 


Введение мнимой единицы позволяет расширить поле вещественных чисел до поля комплексных чисел. Одной из причин введения мнимой единицы является то, что не каждое полиномиальное уравнение 

Исторически мнимая единица сначала была введена для решения вещественного кубического уравнения: при наличии трёх вещественных корней для получения двух из них формула Кардано требовала извлечения квадратных корней из отрицательных чисел.
Вплоть до конца XIX века наряду с символом 



- числа i и −i являются одновременно противоположными и обратными: последнее верно потому, что произведение этих чисел равно 1;
- i и −i комплексно сопряжены, так что их сумма (ноль) и произведение (единица) вещественны одновременно (свойства сопряжённых чисел).
Термин «мнимая единица» может употребляться не только для комплексных чисел, но и для их обобщений[⇨].
Степени мнимой единицы
Степени 
что может быть записано для любой степени в виде:
где n — любое целое число.
Отсюда: 
Возведение в комплексную степень является многозначной функцией. Например, таковой является величина 

где
При 

Также верно, что 
Факториал
Факториал мнимой единицы i можно определить как значение гамма-функции от аргумента 1 + i:
Также
[5]
потому что |i!|2 = i! i! = i! (i)! = Γ(1 + i) Γ(1 − i), что по рекуррентному соотношению гамма-функции можно переписать как i Γ(i) Γ(1 − i), а затем по формуле дополнения Эйлера — как iπsin πi = πsinh π.
Корни из мнимой единицы
Корни квадратные из мнимой единицы
Корни кубические из мнимой единицы (вершины треугольника)
В поле комплексных чисел корень n-й степени имеет n значений. На комплексной плоскости корни из мнимой единицы находятся в вершинах правильного n-угольника, вписанного в окружность с единичным радиусом.
В частности, 
![{displaystyle {{sqrt[{3}]{i}}}=left{-i;~{frac {i+{sqrt {3}}}{2}};~{frac {i-{sqrt {3}}}{2}}right}.}](https://wikimedia.org/api/rest_v1/media/math/render/svg/8c28384d5b7beccaadae3e9c5395e15c82c1d6b1)
Также корни из мнимой единицы могут быть представлены в показательном виде:
Иные мнимые единицы
В конструкции удвоения по Кэли — Диксону или в рамках алгебры по Клиффорду «мнимых единиц расширения» может быть несколько. Но в этом случае могут возникать делители нуля и иные свойства, отличные от свойств комплексного «i».
Например, в теле кватернионов три антикоммутативных мнимых единицы, а также имеется бесконечно много решений уравнения 
К вопросу об интерпретации и названии
Гаусс утверждал также, что если бы величины 1, −1 и √−1 назывались соответственно не положительной, отрицательной и мнимой единицей, а прямой, обратной и побочной, то у людей не создавалось бы впечатления, что с этими числами связана какая-то мрачная тайна. По словам Гаусса, геометрическое представление дает истинную метафизику мнимых чисел в новом свете. Именно Гаусс ввел термин «комплексные числа» (в противоположность «мнимым числам» Декарта) и использовал для обозначения √−1 символ i.Морис Клайн, «Математика. Утрата определённости». Глава VII. Нелогичное развитие: серьёзные трудности на пороге XIX в.
Обозначения
Обычное обозначение — 


В языке программирования Python мнимая единица записывается как 1j.
В языке программирования Wolfram Language мнимая единица записывается как 𝕚.
См.также
- Дуальные числа и двойные числа
- Комплексный анализ
- Кватернион
- Гиперкомплексное число
Примечания
- ↑ Комплексное число // Большая российская энциклопедия : [в 35 т.] / гл. ред. Ю. С. Осипов. — М. : Большая российская энциклопедия, 2004—2017.
- ↑ Мнимая единица // Математическая энциклопедия (в 5 томах). — М.: Советская Энциклопедия, 1982. — Т. 3. — С. 708.
- ↑ Зайцев В. В., Рыжков В. В., Сканави М. И. Элементарная математика. Повторительный курс. — Издание третье, стереотипное. — М.: Наука, 1976. — С. 49. — 591 с.
- ↑ Корн Г., Корн Т. Справочник по математике (для научных работников и инженеров). — 2-е изд. — М.: Наука, 1970. — С. 33. — 720 с.
- ↑ «abs(i!) Архивная копия от 6 июля 2015 на Wayback Machine», WolframAlpha.
Ссылки
- Мнимая единица // Большая советская энциклопедия : [в 30 т.] / гл. ред. А. М. Прохоров. — 3-е изд. — М. : Советская энциклопедия, 1969—1978.
Эта страница в последний раз была отредактирована 16 июля 2022 в 15:27.
Как только страница обновилась в Википедии она обновляется в Вики 2.
Обычно почти сразу, изредка в течении часа.




































![{displaystyle {sqrt[{ni}]{x}}=cos left({frac {ln x}{n}}right)-isin left({frac {ln x}{n}}right)~.}](https://wikimedia.org/api/rest_v1/media/math/render/svg/85698bb8407b1e6c4da720b6ea4e1838624d79e3)






![{displaystyle {begin{aligned}x^{2}-y^{2}&=0\[3mu]2xy&=1.end{aligned}}}](https://wikimedia.org/api/rest_v1/media/math/render/svg/920fdcc901545bfaa91d0654462007376c1b732b)











 или
или 













 [1]
[1]
 [2]
[2]

![sqrt[3]{i } = left{-i; frac{i + {sqrt{3}}}{2}; frac{i - {sqrt{3}}}{2} right}](https://dic.academic.ru/dic.nsf/ruwiki/0e0b4d348e4638bcc7af6cf45c7f8848.png)






















